Publications (en anglais)
-
Articles scientifiques
-
Livres et ouvrages collectifs
-
Mémoires
Voir aussi les profiles sur
Publons,
Orcid ou
Google Scholar.
1. Articles scientifiques
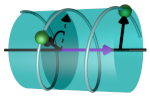
 | Protein-membrane interactions with a twist
Jordan Klein, Loréne Schad, Thérèse E. Malliavin, Martin Michael Müller |
Résumé
Soft Matter, 21: 4336, 2025.
 | Conformational Space of the Translocation Domain of Botulinum Toxin: Atomistic Modeling and Mesoscopic
Description of the Coiled-Coil Helix Bundle
Alexandre Delort, Grazia Cottone, Thérèse E. Malliavin, Martin Michael Müller |
Résumé
Int. J. Mol. Sci., 25: 2481, 2024.
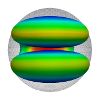 | Flexoelectric fluid membrane vesicles in spherical confinement
Niloufar Abtahi, Lila Bouzar, Nadia Saidi-Amroun, Martin Michael Müller |
Résumé
Plus d'infos
EPL, 131(1): 18001, 2020. Cf. aussi arXiv:2006.04475.
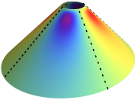 | Isometric bending requires local constraints on free edges
Jemal Guven, Martin Michael Müller, Pablo Vázquez-Montejo |
While the shape equations describing the equilibrium of an unstretchable thin sheet that is free
to bend are known, the boundary conditions that supplement these equations on free edges have remained elusive.
Intuitively, unstretchability is captured by a constraint on the metric within the bulk. Naïvely one would then
guess that this constraint is enough to ensure that the deformations determining the boundary conditions on these
edges respect the isometry constraint. If matters were this simple, unfortunately, it would imply unbalanced torques
(as well as forces) along the edge unless manifestly unphysical constraints are met by the boundary geometry. In this
article, we identify the source of the problem: not only the local arc-length but also the geodesic curvature need to
be constrained explicitly on all free edges. We derive the boundary conditions which follow. In contrast to conventional
wisdom, there is no need to introduce boundary layers. This framework is applied to isolated conical defects, both
with deficit as well, but more briefly, as surplus angles. Using these boundary conditions, we show that the lateral
tension within a circular cone of fixed radius is equal but opposite to the radial compression, and independent of
the deficit angle itself. We proceed to examine the effect of an oblique outer edge on this cone perturbatively
demonstrating that both the correction to the geometry as well as the stress distribution in the cone kicks in at
second order in the eccentricity of the edge.
 Fermer
Plus d'infos Fermer
Plus d'infos
Math. Mech. Solids, 24: 4051, 2019. Cf. aussi arXiv:1904.05855.
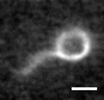 | Helical Superstructure of Intermediate Filaments
Lila Bouzar, Martin Michael Müller, René Messina, Bernd Nöding, Sarah Köster, Hervé Mohrbach, Igor M. Kulić |
Résumé
Plus d'infos
Phys. Rev. Lett., 122: 098101, 2019. Cf. aussi arXiv:1803.04691.
 | Vesicle dynamics in confined steady and harmonically modulated Poiseuille flows
Zakaria Boujja, Chaouqi Misbah, Hamid Ez-Zahraouy, Abdelilah Benyoussef, Thomas John, Christian Wagner, Martin Michael Müller |
We present a numerical study of the time-dependent motion of a membrane vesicle in a
channel under an imposed flow. In a Poiseuille flow the shape of the vesicle depends on the flow strength,
the mechanical properties of the membrane, and the width of the channel. In a wide parameter region, the
emerging snaking shape shows an oscillatory motion like a swimmer flagella even though the flow is
stationary. We quantify this behavior by the amplitude and frequency of the oscillations of the vesicle's
center of mass. The influence of an amplitude modulation of the imposed flow on the dynamics and shape of
the snaking vesicle is also investigated. We find that this modulation---when sufficiently small---induces
a modulation in amplitude and frequency of the center of mass of the snaking vesicle. For large
modulation amplitudes transitions to static shapes are observed.
 Fermer Fermer
Phys. Rev. E, 98: 043111, 2018. Cf. aussi arXiv:1810.04500.
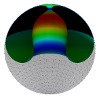 | Confining a fluid membrane vesicle of toroidal topology in an adhesive hard sphere
Lila Bouzar, Ferhat Menas, Martin Michael Müller |
Résumé
Plus d'infos
IOP Conf. Series: MSE, 186: 012021, 2017.
 | Squeezed helical elastica
Lila Bouzar, Martin Michael Müller, Pierre Gosselin, Igor M. Kulić, Hervé Mohrbach |
Résumé
Plus d'infos
Eur. Phys. J. E, 39: 114, 2016. Cf. aussi arXiv:1606.03611.
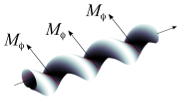 | How bio-filaments twist membranes
Julien Fierling, Albert Johner, Igor M. Kulić, Hervé Mohrbach, Martin Michael Müller |
Résumé
Soft Matter, 12: 5747, 2016.
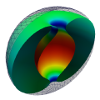 | Toroidal membrane vesicles in spherical confinement
Lila Bouzar, Ferhat Menas, Martin Michael Müller |
Résumé
Plus d'infos
Phys. Rev. E, 92: 032721, 2015. Cf. aussi arXiv:1509.00765.
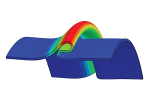 | Non-linear buckling and symmetry breaking of a soft elastic sheet sliding on a cylindrical substrate
Norbert Stoop, Martin Michael Müller |
Résumé
Plus d'infos
Int. J. Non-Linear Mech., 75: 115, 2015. Cf. aussi arXiv:1503.05030.
 | Crunching Biofilament Rings
Julien Fierling, Martin Michael Müller, Hervé Mohrbach, Albert Johner, Igor M. Kulić |
Résumé
Plus d'infos
Europhys. Lett., 107(6): 68002, 2014. Cf. aussi arXiv:1408.6787.
 | Confotronic dynamics of tubular filaments
Osman Kahraman, Hervé Mohrbach, Martin Michael Müller, Igor M. Kulić |
Résumé
Plus d'infos
Soft Matter, 10(16): pp. 2836-2847, 2014. Cf. aussi arXiv:1312.3106.
 | Whirling skirts and rotating cones
Jemal Guven, J. A. Hanna, Martin Michael Müller |
Résumé
New J. Phys., 15: 113055, 2013. Cf. aussi arXiv:1306.2619.
 | Myotubularin and PtdIns3P remodel the sarcoplasmic reticulum in muscle in vivo
Leonela Amoasii, Karim Hnia, Gaëtan Chicanne, Andreas Brech, Belinda Simone Cowling, Martin Michael Müller, Yannick Schwab, Pascale Koebel, Arnaud Ferry, Bernard Payrastre, Jocelyn Laporte |
Résumé
J. Cell Sci., 126(8): 1806, 2013.
 | Dipoles in thin sheets
Jemal Guven, J. A. Hanna, Osman Kahraman, Martin Michael Müller |
A flat elastic sheet may contain pointlike conical singularities that carry a metrical "charge" of Gaussian curvature. Adding such elementary defects to a sheet allows one to make many shapes, in a manner broadly
analogous to the familiar multipole construction in electrostatics. However, here the underlying field theory is non-linear,
and superposition of intrinsic defects is non-trivial as it must respect the immersion of the resulting surface in three
dimensions. We consider a "charge-neutral" dipole composed of two conical singularities of opposite sign.
Unlike the relatively simple electrostatic case, here there are two distinct stable minima and an infinity of unstable equilibria.
We determine the shapes of the minima and evaluate their energies in the thin-sheet regime where bending dominates
over stretching. Our predictions are in surprisingly good agreement with experiments on paper sheets.
 Fermer
Plus d'infos Fermer
Plus d'infos
Eur. Phys. J. E, 36: 106, 2013. Cf. aussi arXiv:1212.3262.
 | Fluid membrane vesicles in confinement
Osman Kahraman, Norbert Stoop, Martin Michael Müller |
We numerically study the morphology of fluid membrane vesicles with prescribed volume and surface area in confinement.
For spherical confinement we observe axisymmetric invaginations that transform into ellipsoidal invaginations a the area of the
vesicle is increased, followed by a transition into stomatocyte-like shapes.
We provide a detailed analysis of the axisymmetric shapes and investigate the effect of the spontaneous curvature of the membrane
as a possible mechanism for shape regulation. We show that the observed morphologies are stable under small geometric deformations
of the confinement. The results could help to understand the role of mechanics in the complex folding patterns of biological membranes.
 Fermer
Plus d'infos Fermer
Plus d'infos
New J. Phys., 14: 095021, 2012.
 | Petal shapes of sympetaleous flowers: the interplay between growth, geometry and elasticity
Martine Ben Amar, Martin Michael Müller, Miguel Trejo |
Résumé
Plus d'infos
New J. Phys., 14: 085014, 2012. Choisi pour les Highlights of 2012.
 | Morphogenesis of membrane invaginations in spherical confinement
Osman Kahraman, Norbert Stoop, Martin Michael Müller |
Résumé
Plus d'infos
Europhys. Lett., 97(6): 68008, 2012. Cf. aussi arXiv:1201.2518.
 | Conical instabilities on paper
Jemal Guven, Martin Michael Müller, Pablo Vázquez-Montejo |
Résumé
Plus d'infos
J. Phys. A: Math. Theor., 45(1): 015203, 2012. Cf. aussi arXiv:1107.5008.
 | Interface-mediated interactions: Entropic forces of curved membranes
Pierre Gosselin, Hervé Mohrbach, Martin Michael Müller |
Résumé
Plus d'infos
Phys. Rev. E, 83(5): 051921, 2011. Cf. aussi arXiv:1011.1221.
 | Self-Contact and Instabilities in the Anisotropic Growth of Elastic Membranes
Norbert Stoop, Falk K. Wittel, Martine Ben Amar, Martin Michael Müller, Hans J. Herrmann |
Résumé
Plus d'infos
Phys. Rev. Lett., 105(6): 068101, 2010. Cf. aussi arXiv:1007.1871.
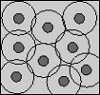 | Cell Model Approach to Membrane Mediated Protein Interactions
Martin Michael Müller, Markus Deserno |
Résumé
Plus d'infos
Prog. Theor. Phys. Suppl., 184: pp. 351-363, 2010.
 | Hamiltonian formulation of surfaces with constant Gaussian curvature
Miguel Trejo, Martine Ben Amar, Martin Michael Müller |
Dirac's method for constrained Hamiltonian systems is used to describe surfaces of constant Gaussian curvature. A geometrical free energy, for which these surfaces are equilibrium states, is introduced and interpreted as an action. An equilibrium surface can then be generated by the evolution of a closed space curve.
Since the underlying action depends on second derivatives, the velocity of the curve and its conjugate momentum must be included in the set of phase space variables. Furthermore, the action is linear in the acceleration of the curve and possesses a local symmetry---reparametrization invariance---which implies primary constraints in the canonical formalism. These constraints are incorporated into the Hamiltonian through Lagrange multiplier functions, that are identified as the components of the acceleration of the curve. The formulation leads to four first order partial differential equations, one for each canonical variable.
With the appropriate choice of parametrization only one of these equations has to be solved to obtain the surface which is swept out by the evolving space curve. To illustrate the formalism, several evolutions of pseudospherical surfaces are discussed.
 Fermer
Plus d'infos Fermer
Plus d'infos
J. Phys. A: Math. Theor., 42(42): 425204, 2009.
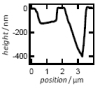 | Local Membrane Mechanics of Pore-Spanning Bilayers
Ingo Mey, Milena Stephan, Eva K. Schmitt, Martin Michael Müller, Martine Ben Amar, Claudia Steinem, Andreas Janshoff |
Résumé
Plus d'infos
J. Am. Chem. Soc., 131(20): pp. 7031-7039, 2009.
 | Elasticity Mapping of Pore-Suspending Native Cell Membranes
Bärbel Lorenz, Ingo Mey, Siegfried Steltenkamp, Tamir Fine, Christina Rommel, Martin Michael Müller, Alexander Maiwald, Joachim Wegener, Claudia Steinem, Andreas Janshoff |
Résumé
Plus d'infos
Small, 5(7): pp. 832-838, 2009.
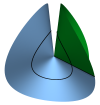 | Conical Defects in Growing Sheets
Martin Michael Müller, Martine Ben Amar, Jemal Guven |
Résumé
Plus d'infos
Phys. Rev. Lett., 101(15): 156104, 2008. Cf. aussi arXiv:0807.1814.
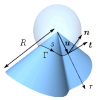 | How paper folds: bending with local constraints
Jemal Guven, Martin Michael Müller |
Résumé
Plus d'infos
J. Phys. A: Math. Theor., 41(5): 055203, 2008. Cf. aussi arXiv:0712.0978.
 | Contact lines for fluid surface adhesion
Markus Deserno, Martin Michael Müller, Jemal Guven |
When a fluid surface adheres to a substrate, the location of the
contact line adjusts in order to minimize the overall energy. This
adhesion balance implies boundary conditions which depend on the
characteristic surface deformation energies. We develop a general
geometrical framework within which these conditions can be
systematically derived.
We treat both adhesion to a rigid substrate as well as adhesion
between two fluid surfaces, and illustrate our general results for
several important Hamiltonians involving both curvature and
curvature gradients. Some of these have previously been studied
using very different techniques, others are to our knowledge new.
What becomes clear in our approach is that, except for capillary
phenomena, these boundary conditions are not the manifestation
of a local force balance, even if the concept of surface stress is
properly generalized. Hamiltonians containing higher order surface
derivatives are not just sensitive to boundary translations but also
notice changes in slope or even curvature.
Both the necessity and the functional form of the corresponding
additional contributions follow readily from our treatment.
 Fermer
Plus d'infos Fermer
Plus d'infos
Phys. Rev. E, 76(1): 011605, 2007. Cf. aussi cond-mat/0703019.
 | Balancing torques in membrane-mediated interactions: Exact results and
numerical illustrations
Martin Michael Müller, Markus Deserno, Jemal Guven |
Torques on interfaces can be described by a divergence-free tensor
which is fully encoded in the geometry. This tensor consists of two
terms, one originating in the couple of the stress, the other capturing
an intrinsic contribution due to curvature. In analogy to the description
of forces in terms of a stress tensor, the torque on a particle can be
expressed as a line integral along any contour surrounding the particle.
Interactions between particles mediated by a fluid membrane are studied
within this framework. In particular, torque balance places a strong
constraint on the shape of the membrane. Symmetric two-particle
configurations admit simple analytical expressions which are valid
in the fully nonlinear regime; in particular, the problem may be
solved exactly in the case of two membrane-bound parallel cylinders.
This apparently simple system provides some flavor of the remarkably
subtle nonlinear behavior associated with membrane-mediated interactions.
 Fermer
Plus d'infos Fermer
Plus d'infos
Phys. Rev. E, 76(1): 011921, 2007. Cf. aussi cond-mat/0702340.
 | Aggregation and vesiculation of membrane proteins by curvature-mediated
interactions
Benedict J. Reynwar, Gregoria Illya, Vagelis A. Harmandaris, Martin Michael Müller, Kurt Kremer, Markus Deserno |
Résumé
Plus d'infos
Nature 447(7143): pp. 461-464, 2007.
 | How to determine local elastic properties of lipid bilayer membranes
from atomic-force-microscope measurements: A theoretical analysis
Davood Norouzi, Martin Michael Müller, Markus Deserno |
Résumé
Plus d'infos
Phys. Rev. E, 74(6): 061914, 2006. Cf. aussi cond-mat/0602662.
 | Mechanical Properties of Pore-Spanning Lipid Bilayers Probed by Atomic Force Microscopy
Siegfried Steltenkamp, Martin Michael Müller, Markus Deserno, Christian Hennesthal, Claudia Steinem, Andreas Janshoff |
Résumé
Plus d'infos
Biophys. J., 91(1): pp. 217-226, 2006.
 | Interface mediated interactions between particles -- a geometrical approach
Martin Michael Müller, Markus Deserno, Jemal Guven |
Résumé
Plus d'infos
Phys. Rev. E, 72(6): 061407, 2005. Cf. aussi cond-mat/0506019.
 | Geometry of surface-mediated interactions
Martin Michael Müller, Markus Deserno, Jemal Guven |
Résumé
Plus d'infos
Europhys. Lett., 69(3): pp. 482-488, 2005. Cf. aussi cond-mat/0409043.
2. Livres et ouvrages collectifs
3. Mémoires
-
Theoretical examinations of interface mediated interactions between colloidal particles,
mémoire (2004).
-
Theoretical studies of fluid membrane mechanics, thèse de doctorat (2007).
-
Symmetry breaking in bioelasticity, thèse d'habilitation à diriger des recherches (2015).
|


