Publications (en anglais)
-
Articles scientifiques
-
Livres
-
Mémoires
Voir aussi les profiles sur
Publons,
Orcid ou
Google Scholar.
1. Articles scientifiques
 | Conformational Space of the Translocation Domain of Botulinum Toxin: Atomistic Modeling and Mesoscopic
Description of the Coiled-Coil Helix Bundle
Alexandre Delort, Grazia Cottone, Thérèse E. Malliavin, Martin Michael Müller |
Résumé
Int. J. Mol. Sci., 25: 2481, 2024.
 | Flexoelectric fluid membrane vesicles in spherical confinement
Niloufar Abtahi, Lila Bouzar, Nadia Saidi-Amroun, Martin Michael Müller |
Résumé
Plus d'infos
EPL, 131(1): 18001, 2020. Cf. aussi arXiv:2006.04475.
 | Isometric bending requires local constraints on free edges
Jemal Guven, Martin Michael Müller, Pablo Vázquez-Montejo |
Résumé
Plus d'infos
Math. Mech. Solids, 24: 4051, 2019. Cf. aussi arXiv:1904.05855.
 | Helical Superstructure of Intermediate Filaments
Lila Bouzar, Martin Michael Müller, René Messina, Bernd Nöding, Sarah Köster, Hervé Mohrbach, Igor M. Kulić |
Résumé
Plus d'infos
Phys. Rev. Lett., 122: 098101, 2019. Cf. aussi arXiv:1803.04691.
 | Vesicle dynamics in confined steady and harmonically modulated Poiseuille flows
Zakaria Boujja, Chaouqi Misbah, Hamid Ez-Zahraouy, Abdelilah Benyoussef, Thomas John, Christian Wagner, Martin Michael Müller |
Résumé
Phys. Rev. E, 98: 043111, 2018. Cf. aussi arXiv:1810.04500.
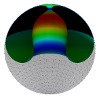 | Confining a fluid membrane vesicle of toroidal topology in an adhesive hard sphere
Lila Bouzar, Ferhat Menas, Martin Michael Müller |
Résumé
Plus d'infos
IOP Conf. Series: MSE, 186: 012021, 2017.
 | Squeezed helical elastica
Lila Bouzar, Martin Michael Müller, Pierre Gosselin, Igor M. Kulić, Hervé Mohrbach |
We theoretically study the conformations of a helical semi-flexible filament
confined to a two-dimensional surface. This squeezed helix exhibits a variety of unexpected shapes
resembling circles, waves or spirals depending on the material parameters. We explore the conformation
space in detail and show that the shapes can be understood as the mutual elastic interaction of
conformational quasi-particles. Our theoretical results are potentially useful to
determine the material parameters of such helical filaments in an experimental setting.
 Fermer
Plus d'infos Fermer
Plus d'infos
Eur. Phys. J. E, 39: 114, 2016. Cf. aussi arXiv:1606.03611.
 | How bio-filaments twist membranes
Julien Fierling, Albert Johner, Igor M. Kulić, Hervé Mohrbach, Martin Michael Müller |
We study the deformations of a fluid membrane imposed by adhering stiff
bio-filaments due to the torques they apply. In the limit of small deformations, we
derive a general expression for the energy and the deformation field of the membrane.
This expression is specialised to different important cases including closed and helical
bio-filaments. In particular, we analyse interface-mediated interactions and membrane
wrapping when the filaments apply a local torque distribution on a tubular membrane.
 Fermer Fermer
Soft Matter, 12: 5747, 2016.
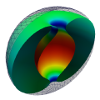 | Toroidal membrane vesicles in spherical confinement
Lila Bouzar, Ferhat Menas, Martin Michael Müller |
Résumé
Plus d'infos
Phys. Rev. E, 92: 032721, 2015. Cf. aussi arXiv:1509.00765.
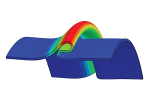 | Non-linear buckling and symmetry breaking of a soft elastic sheet sliding on a cylindrical substrate
Norbert Stoop, Martin Michael Müller |
Résumé
Plus d'infos
Int. J. Non-Linear Mech., 75: 115, 2015. Cf. aussi arXiv:1503.05030.
 | Crunching Biofilament Rings
Julien Fierling, Martin Michael Müller, Hervé Mohrbach, Albert Johner, Igor M. Kulić |
Résumé
Plus d'infos
Europhys. Lett., 107(6): 68002, 2014. Cf. aussi arXiv:1408.6787.
 | Confotronic dynamics of tubular filaments
Osman Kahraman, Hervé Mohrbach, Martin Michael Müller, Igor M. Kulić |
Tubular lattices are ubiquitous in nature and technology. Microtubules and nanotubes of all
kinds act as important pillars of biological cells and the man-made nano-world. We show that
when prestress is introduced in such structures, localized conformational quasiparticles emerge and
govern the collective shape dynamics of the lattice. When coupled via cooperative interactions these
quasiparticles form larger-scale quasipolymer superstructures exhibiting collective dynamic modes
and giving rise to a hallmark behavior radically different from semiflexible beams.
 Fermer
Plus d'infos Fermer
Plus d'infos
Soft Matter, 10(16): pp. 2836-2847, 2014. Cf. aussi arXiv:1312.3106.
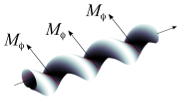
 | Whirling skirts and rotating cones
Jemal Guven, J. A. Hanna, Martin Michael Müller |
Steady, dihedrally symmetric patterns with sharp peaks may be observed on a spinning skirt, lagging behind the material flow of the fabric. These qualitative features are captured with a minimal model of traveling waves on an inextensible, flexible, generalized-conical sheet rotating about a fixed axis. Conservation laws are used to reduce the dynamics to a quadrature describing a particle in a three-parameter family of potentials. One parameter is associated with the stress in the sheet, aNoether is the current associated with rotational invariance, and the third is a Rossby number which indicates the relative strength of Coriolis forces. Solutions are quantized by enforcing a topology appropriate to a skirt and a particular choice of dihedral symmetry. A perturbative analysis of nearly axisymmetric cones shows that Coriolis effects are essential in establishing skirt-like solutions. Fully non-linear solutions with three-fold symmetry are presented which bear a suggestive resemblance to the observed patterns.
 Fermer Fermer
New J. Phys., 15: 113055, 2013. Cf. aussi arXiv:1306.2619.
 | Myotubularin and PtdIns3P remodel the sarcoplasmic reticulum in muscle in vivo
Leonela Amoasii, Karim Hnia, Gaëtan Chicanne, Andreas Brech, Belinda Simone Cowling, Martin Michael Müller, Yannick Schwab, Pascale Koebel, Arnaud Ferry, Bernard Payrastre, Jocelyn Laporte |
Résumé
J. Cell Sci., 126(8): 1806, 2013.
 | Dipoles in thin sheets
Jemal Guven, J. A. Hanna, Osman Kahraman, Martin Michael Müller |
A flat elastic sheet may contain pointlike conical singularities that carry a metrical "charge" of Gaussian curvature. Adding such elementary defects to a sheet allows one to make many shapes, in a manner broadly
analogous to the familiar multipole construction in electrostatics. However, here the underlying field theory is non-linear,
and superposition of intrinsic defects is non-trivial as it must respect the immersion of the resulting surface in three
dimensions. We consider a "charge-neutral" dipole composed of two conical singularities of opposite sign.
Unlike the relatively simple electrostatic case, here there are two distinct stable minima and an infinity of unstable equilibria.
We determine the shapes of the minima and evaluate their energies in the thin-sheet regime where bending dominates
over stretching. Our predictions are in surprisingly good agreement with experiments on paper sheets.
 Fermer
Plus d'infos Fermer
Plus d'infos
Eur. Phys. J. E, 36: 106, 2013. Cf. aussi arXiv:1212.3262.
 | Fluid membrane vesicles in confinement
Osman Kahraman, Norbert Stoop, Martin Michael Müller |
Résumé
Plus d'infos
New J. Phys., 14: 095021, 2012.
 | Petal shapes of sympetaleous flowers: the interplay between growth, geometry and elasticity
Martine Ben Amar, Martin Michael Müller, Miguel Trejo |
Résumé
Plus d'infos
New J. Phys., 14: 085014, 2012. Choisi pour les Highlights of 2012.
 | Morphogenesis of membrane invaginations in spherical confinement
Osman Kahraman, Norbert Stoop, Martin Michael Müller |
Résumé
Plus d'infos
Europhys. Lett., 97(6): 68008, 2012. Cf. aussi arXiv:1201.2518.
 | Conical instabilities on paper
Jemal Guven, Martin Michael Müller, Pablo Vázquez-Montejo |
The stability of the fundamental defects of an unstretchable flat sheet is examined.
This involves expanding the bending energy to second order in deformations about the
defect. The modes of deformation occur as eigenstates of a fourth-order linear differential
operator. Unstretchability places a global linear constraint on these modes. Conical
defects with a surplus angle exhibit an infinite number of states. If this angle is below a
critical value, these states possess an n-fold symmetry labeled by an integer, n ≥ 2. A
nonlinear stability analysis shows that the 2-fold ground state is stable, whereas excited
states possess 2(n - 2) unstable modes which come in even and odd pairs.
 Fermer
Plus d'infos Fermer
Plus d'infos
J. Phys. A: Math. Theor., 45(1): 015203, 2012. Cf. aussi arXiv:1107.5008.
 | Interface-mediated interactions: Entropic forces of curved membranes
Pierre Gosselin, Hervé Mohrbach, Martin Michael Müller |
Résumé
Plus d'infos
Phys. Rev. E, 83(5): 051921, 2011. Cf. aussi arXiv:1011.1221.
 | Self-Contact and Instabilities in the Anisotropic Growth of Elastic Membranes
Norbert Stoop, Falk K. Wittel, Martine Ben Amar, Martin Michael Müller, Hans J. Herrmann |
Résumé
Plus d'infos
Phys. Rev. Lett., 105(6): 068101, 2010. Cf. aussi arXiv:1007.1871.
 | Cell Model Approach to Membrane Mediated Protein Interactions
Martin Michael Müller, Markus Deserno |
Résumé
Plus d'infos
Prog. Theor. Phys. Suppl., 184: pp. 351-363, 2010.
 | Hamiltonian formulation of surfaces with constant Gaussian curvature
Miguel Trejo, Martine Ben Amar, Martin Michael Müller |
Dirac's method for constrained Hamiltonian systems is used to describe surfaces of constant Gaussian curvature. A geometrical free energy, for which these surfaces are equilibrium states, is introduced and interpreted as an action. An equilibrium surface can then be generated by the evolution of a closed space curve.
Since the underlying action depends on second derivatives, the velocity of the curve and its conjugate momentum must be included in the set of phase space variables. Furthermore, the action is linear in the acceleration of the curve and possesses a local symmetry---reparametrization invariance---which implies primary constraints in the canonical formalism. These constraints are incorporated into the Hamiltonian through Lagrange multiplier functions, that are identified as the components of the acceleration of the curve. The formulation leads to four first order partial differential equations, one for each canonical variable.
With the appropriate choice of parametrization only one of these equations has to be solved to obtain the surface which is swept out by the evolving space curve. To illustrate the formalism, several evolutions of pseudospherical surfaces are discussed.
 Fermer
Plus d'infos Fermer
Plus d'infos
J. Phys. A: Math. Theor., 42(42): 425204, 2009.
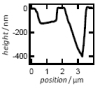 | Local Membrane Mechanics of Pore-Spanning Bilayers
Ingo Mey, Milena Stephan, Eva K. Schmitt, Martin Michael Müller, Martine Ben Amar, Claudia Steinem, Andreas Janshoff |
The mechanical behavior of lipid bilayers spanning the pores of highly ordered porous silicon substrates was studied by local indentation experiments as a function of surface functionalization, lipid composition, solvent content, indentation velocity, and pore radius. Solvent-containing nanoblack lipid membranes (nano-BLMs) as well as solvent-free pore-spanning bilayers were imaged by fluorescence and atomic force microscopy prior to force curve acquisition, which allows distinguishing between membrane-covered and uncovered pores. Force indentation curves on pore-spanning bilayers attached to functionalized hydrophobic porous silicon substrates reveal a predominately linear response that is mainly attributed to prestress in the membranes. This is in agreement with the observation that indentation leads to membrane lysis well below 5% area dilatation. However, membrane bending and lateral tension dominates over
prestress and stretching if solvent-free supported membranes obtained from spreading giant liposomes on hydrophilic porous silicon are indented.
 Fermer
Plus d'infos Fermer
Plus d'infos
J. Am. Chem. Soc., 131(20): pp. 7031-7039, 2009.
 | Elasticity Mapping of Pore-Suspending Native Cell Membranes
Bärbel Lorenz, Ingo Mey, Siegfried Steltenkamp, Tamir Fine, Christina Rommel, Martin Michael Müller, Alexander Maiwald, Joachim Wegener, Claudia Steinem, Andreas Janshoff |
The mechanics of cellular membranes is governed by a non-equilibrium composite framework
consisting of the semiflexible filamentous cytoskeleton and extracellular matrix proteins linked to
the lipid bilayer. While elasticity information of plasma membranes has mainly been obtained from
whole cell analysis, techniques that allow to address local mechanical properties of cell
membranes are desirable to learn how their lipid and protein composition is reflected in the elastic
behavior on local length scales. Here, we introduce an approach based on basolateral
membranes of polar epithelial Madin-Darby canine kidney (MDCK) II cells, prepared on a highly ordered porous substrate that
allows elastic mapping on a submicrometer length scale. A strong correlation between the
density of actin filaments and the measured membrane elasticity is found. Spatially resolved indentation experiments carried out with atomic force and fluorescence microscope permit to relate the supramolecular structure to the elasticity of cellular membranes. It is shown that the elastic response of the pore-spanning cell membranes is governed by the local bending modules rather than the lateral tension.
 Fermer
Plus d'infos Fermer
Plus d'infos
Small, 5(7): pp. 832-838, 2009.
 | Conical Defects in Growing Sheets
Martin Michael Müller, Martine Ben Amar, Jemal Guven |
Résumé
Plus d'infos
Phys. Rev. Lett., 101(15): 156104, 2008. Cf. aussi arXiv:0807.1814.
 | How paper folds: bending with local constraints
Jemal Guven, Martin Michael Müller |
Résumé
Plus d'infos
J. Phys. A: Math. Theor., 41(5): 055203, 2008. Cf. aussi arXiv:0712.0978.
 | Contact lines for fluid surface adhesion
Markus Deserno, Martin Michael Müller, Jemal Guven |
When a fluid surface adheres to a substrate, the location of the
contact line adjusts in order to minimize the overall energy. This
adhesion balance implies boundary conditions which depend on the
characteristic surface deformation energies. We develop a general
geometrical framework within which these conditions can be
systematically derived.
We treat both adhesion to a rigid substrate as well as adhesion
between two fluid surfaces, and illustrate our general results for
several important Hamiltonians involving both curvature and
curvature gradients. Some of these have previously been studied
using very different techniques, others are to our knowledge new.
What becomes clear in our approach is that, except for capillary
phenomena, these boundary conditions are not the manifestation
of a local force balance, even if the concept of surface stress is
properly generalized. Hamiltonians containing higher order surface
derivatives are not just sensitive to boundary translations but also
notice changes in slope or even curvature.
Both the necessity and the functional form of the corresponding
additional contributions follow readily from our treatment.
 Fermer
Plus d'infos Fermer
Plus d'infos
Phys. Rev. E, 76(1): 011605, 2007. Cf. aussi cond-mat/0703019.
Choisi pour le Virtual Journal of Biological Physics Research.
 | Balancing torques in membrane-mediated interactions: Exact results and
numerical illustrations
Martin Michael Müller, Markus Deserno, Jemal Guven |
Torques on interfaces can be described by a divergence-free tensor
which is fully encoded in the geometry. This tensor consists of two
terms, one originating in the couple of the stress, the other capturing
an intrinsic contribution due to curvature. In analogy to the description
of forces in terms of a stress tensor, the torque on a particle can be
expressed as a line integral along any contour surrounding the particle.
Interactions between particles mediated by a fluid membrane are studied
within this framework. In particular, torque balance places a strong
constraint on the shape of the membrane. Symmetric two-particle
configurations admit simple analytical expressions which are valid
in the fully nonlinear regime; in particular, the problem may be
solved exactly in the case of two membrane-bound parallel cylinders.
This apparently simple system provides some flavor of the remarkably
subtle nonlinear behavior associated with membrane-mediated interactions.
 Fermer
Plus d'infos Fermer
Plus d'infos
Phys. Rev. E, 76(1): 011921, 2007. Cf. aussi cond-mat/0702340.
Choisi pour le Virtual Journal of Biological Physics Research.
 | Aggregation and vesiculation of membrane proteins by curvature-mediated
interactions
Benedict J. Reynwar, Gregoria Illya, Vagelis A. Harmandaris, Martin Michael Müller, Kurt Kremer, Markus Deserno |
Résumé
Plus d'infos
Nature 447(7143): pp. 461-464, 2007.
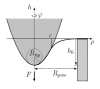 | How to determine local elastic properties of lipid bilayer membranes
from atomic-force-microscope measurements: A theoretical analysis
Davood Norouzi, Martin Michael Müller, Markus Deserno |
Résumé
Plus d'infos
Phys. Rev. E, 74(6): 061914, 2006. Cf. aussi cond-mat/0602662.
Choisi pour le Virtual Journal of Biological Physics Research.
 | Mechanical Properties of Pore-Spanning Lipid Bilayers Probed by Atomic Force Microscopy
Siegfried Steltenkamp, Martin Michael Müller, Markus Deserno, Christian Hennesthal, Claudia Steinem, Andreas Janshoff |
We measure the elastic response of a free-standing lipid membrane to a local indentation by using an atomic force microscope. Starting point is a planar
gold-coated alumina substrate with a chemisorbed 3-mercaptopropionic acid
monolayer displaying circular pores of very well defined and tunable size, over
which bilayers composed of N,N,- dimethyl- N,N,- dioctadecylammonium bromide or
1,2 - dioleoyl - 3 - trimethylammonium - propane chloride were spread.
Centrally indenting these 'nanodrums' with an atomic force microscope tip yields
force-indentation curves, which we quantitatively analyze by solving the
corresponding shape equations of continuum curvature elasticity. Since the
measured response depends in a known way on the system geometry (pore size, tip
radius) and on material parameters (bending modulus, lateral tension), this opens
the possibility to monitor local elastic properties of lipid membranes in a
well-controlled setting.
 Fermer
Plus d'infos Fermer
Plus d'infos
Biophys. J., 91(1): pp. 217-226, 2006.
 | Interface mediated interactions between particles -- a geometrical approach
Martin Michael Müller, Markus Deserno, Jemal Guven |
Résumé
Plus d'infos
Phys. Rev. E, 72(6): 061407, 2005. Cf. aussi cond-mat/0506019.
Choisi pour le Virtual Journal of Biological Physics Research.
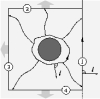 | Geometry of surface-mediated interactions
Martin Michael Müller, Markus Deserno, Jemal Guven |
Résumé
Plus d'infos
Europhys. Lett., 69(3): pp. 482-488, 2005. Cf. aussi cond-mat/0409043.
2. Livres
-
New Trends in the Physics and Mechanics of Biological Systems
Lecture Notes of the Les Houches Summer School, vol. 92 (Oxford University Press, 2011),
éd. par Martine Ben Amar, Alain Goriely, Martin Michael Müller et Leticia Cugliandolo.
Chapitre 9 :
The physics of the cell membrane
Martin Michael Müller et Martine Ben Amar.
3. Mémoires
-
Theoretical examinations of interface mediated interactions between colloidal particles,
mémoire (2004).
-
Theoretical studies of fluid membrane mechanics, thèse de doctorat (2007).
-
Symmetry breaking in bioelasticity, thèse d'habilitation à diriger des recherches (2015).
|


